Chapter 43: Problem 12

Question: Light from a laser enters a glass block at A and emerges at B, as shown in Fig 29 in Chapter 43. The glass block has length $L~=~0.547~{\rm m}$, and a refractive index of n = 1.63. The angle of incidence is $\theta_1~=~24^o$, find the time needed for the light to pass through the block.

Solution:

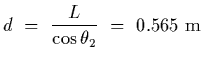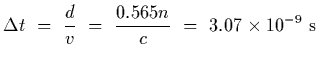$$n_1 \sin\theta_1~=~ n_2 \sin\theta_2$$

from which we find that $\sin\theta_2~=~0.25$ and hence $\cos\theta_2~=~0.968$. The length of the path traveled by the light in the block, and the time to do so, is

$$d~=~{L\over\cos\theta_2}~=~0.565~{\rm m}$$

$$\Delta t~=~{d\over v}~=~{0.565 n\over c}~=~3.07\times 10^{-9}~{\rm s}$$