A. Rajantie
University of Sussex
Defect formation is believed to be relatively well understood
in second-order symmetry breaking phase transitions.
Zurek [1]
has given a simple estimate for the produced defect density,
based on the critical slowing down of the system near a
second-order transition.
If the temperature decreases rapidly enough, the fields fall
out of equilibrium before reaching the critical point,
and the correlation length of the order parameter freezes to
a value
where 
is the time scale related to the the temperature ``quench'',
and 
and 
are the critical exponents related to the diverging
of the correlation length and the relaxation time, respectively:
This freeze-out correlation length gives the average distance between
the defects. In cosmology, one also gets a lower bound for the defect
density from the fact that the correlation length cannot be larger than
the horizon size.
In cosmology, however, the phase transitions are more likely to be related to a ``breakdown'' of local gauge symmetries, i.e. the Higgs mechanism, than a true spontaneous symmetry breakdown. In that case, the direction of the order parameter has no physical meaning and there is no correlation length that would be directly related to the final defect density. Therefore, Zurek's arguments, or even the causality argument, do not necessarily have to apply.
In the absence of any convincing analytical arguments, the only possible
way to understand defect formation is to carry out real-time non-equilibrium
simulations numerically. With M. Hindmarsh, we chose to study the
Abelian Higgs model in the hard thermal loop approximation [2].
The equations of motion can be formulated in a local way by introducing
two extra fields 
and 
,
which depend, in addition to the space-time coordinates, on an
internal ``coordinate'' 
.
We took the initial conditions from a thermal ensemble in the
Coulomb (symmetric) phase, and solved numerically the equations of motion,
decreasing the bare mass parameter smoothly during the simulation so that
eventually the system underwent a phase transition to the Higgs phase.
The rate of change of 
was parameterized by the quench
time-scale .
In the lattice we used, one dimension was much shorter than the others,
but still longer than the correlation lengths. In the final state,
we counted the
number of string defects passing through the system in this short direction.
This procedure was repeated for many different quench rates and initial
configurations.
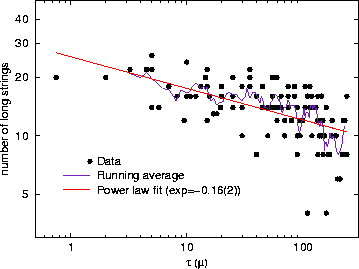
Figure 1:
Number of ``long'' strings, i.e. those that stretch through the short dimension
of the lattice, in the final state as a function of the quench time-scale
.
Our results show that the dependence of the final defect density on
is indeed consistent with a power law, but with a fairly small exponent
(see Fig. 1).
In comparison, the value for a global
theory, i.e. one without gauge fields, is much longer ,
[3]. This shows that the presence of the
gauge field changes essentially this process. It remains an open
question, whether there is a simple physical picture, like the Zurek picture
for global theories, that can explain the density of defects formed in
the transition.