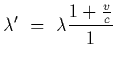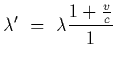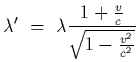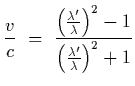

Next: Chapter 42: Problem 14
Up: Waves and Optics, Solutions
Previous: Chapter 42: Problem 11
Question: In the spectrum of quaser 3C9, some of the familiar hydrogen
lines appear but they are shifted so far forward toward the red that their
wavelengths are observed to be 3 times as large as that observed in the
light from hydrogen atoms at rest in the laboratory.
- 1.
- Show that the Doppler equation, which assumes that light behaves like
sound gives a velocity of recession greater than the speed of light.
- 2.
- Assuming that the relative motion of 3C9 and the earth is entirely one
of recession, find the recession speed predicted by the relativistic
Doppler relation.
Solution: If light behaved like sound, we would have that
and for
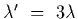 ,
we would need v = 2c.
For light, however, we know that
,
we would need v = 2c.
For light, however, we know that
from which we can show that
for pure recession, and hence we have that v = 0.8c.


Next: Chapter 42: Problem 14
Up: Waves and Optics, Solutions
Previous: Chapter 42: Problem 11
Martin Savage
1999-02-03