
INSTITUTE FOR NUCLEAR THEORY
News
Home | Contact | Search | News archive | Site Map

|
INSTITUTE FOR NUCLEAR THEORY News
Home | Contact | Search | News archive | Site Map |
|||||
The QCD critical point
The QCD phase diagram is a map showing what equilibrium state strongly interacting matter would assume at given temperature and baryon density. The nature of the different phases and the location of the phase boundaries have been intriguing questions for many years.
Soon after the discovery of asymptotic freedom the existence of a new phase of matter consisting of almost free quarks and gluons has been predicted. This phase is now known as the Quark Gluon Plasma (QGP). Early on a description of QCD thermodynamics based on the MIT bag model suggested a first order transition from a gas of hadrons directly into weakly interacting QGP. Since then, however, progress in Lattice QCD and data from RHIC has shown that this had been a rather simplistic picture.
From Lattice QCD we now know rather conclusively that at vanishing baryon density the transition in QCD is an analytic crossover. In addition, the surprisingly strong elliptic flow observed at RHIC suggests that the matter at temperatures up to two times above that crossover is still far from being weakly interacting gas. Rather, it behaves as a strongly coupled liquid.
The reliable theoretical knowledge of the QCD phase diagram coming from Lattice Monte Carlo simulations is severely limited by the sign problem to the regime of zero net baryon density, i.e., zero baryochemical potential μB. The basic question which remains open is whether the transition to QGP always remains a crossover, or turns into a first order transition at sufficiently large μB. If it does turn into a first order transition, as is the case in common liquids, including water, the phase diagram of QCD must possess a critical point (see Figure).
The critical point is a distinct singular feature of the phase diagram which anchors the location of the first order phase transition as well. The discovery of the QCD critical point would fundamentally transform our understanding of the QCD phase diagram, turning it from a semi-quantitative sketch into quantitative and reliable knowledge and guidance to further theoretical and experimental research.
Can the QCD critical point be discovered experimentally? Heavy ion collisions can scan the QCD phase diagram in the T, μB plane by varying the initial collision energy. The critical point has characteristic properties, which manifest themselves when the freeze-out occurs in the proximity of this point. One such property, universal to all critical points, is the anomalous increase of the magnitude of fluctuations which can be detected using event-by-event measurements. Recently, the anomalous properties of the equation of state, which could affect hydrodynamic evolution, and possibly be detected in a heavy-ion collision experiments also received attention.
Figure: The trajectory of the energy scan of the QCD phase diagram (numbers by the circle points indicate initial collision center of mass energy in GeV per nucleon pair). Diamonds are the lattice Monte Carlo predictions for the location of the critical point. The errors are not shown.
The purpose of the INT Program QCD Critical Point held between July 27 and August 24, 2008 was to bring together theorists as well as experimentalists to discuss recent progress and develop strategies to discover the critical point, both theoretically and experimentally.
Determining the location of the critical point of QCD has been a long-standing challenge.
The earliest indications that the QCD phase diagram features a critical point came from
Nambu-Jona-Lasinio models, incorporating physics of spontaneous chiral symmetry breaking. Although the critical point separating crossover from first-order transition appeared as a rather robust feature of these models, the location of the point was clearly and significantly dependent on the model parameters. This fact has been emphasized recently, and discussed during the INT Program. The dependence on model parameters is so strong that by a suitable extreme choice of the model parameters the critical point could be even "dialed off" the phase diagram.
Another interesting question which received renewed attention recently is the sensitivity of the position of the critical point to the physics associated with the 't Hooft's anomalous interaction involving all three relevant flavors of quarks: up, down and strange.
Lattice Monte Carlo is the method of choice when it comes to addressing non-perturbative problems in QCD, such as QCD thermodynamics and phase transitions. The most interesting region in the phase diagram is at a scale of temperatures and densities where asymptotic freedom is not yet operative and QCD is strongly coupled. Unfortunately the Lattice Monte Carlo approach is not yet suitable to study QCD at non-zero chemical potential due to the well-known sign problem. Much of the discussions at the INT Program focused on current methods of addressing this problem.
In particular, the Taylor expansion methods received significant attention. It is clear that Taylor coefficients of the QCD thermodynamic functions such as pressure or its derivatives contain information about the location of the critical point. How much, and what is the best way to extract this information, remains an open question, that has been intensively discussed during the program. Higher order cumulants (derivatives of pressure with respect to chemical potential) are a promising source of such information. Pade approximation techniques, which so far have not been applied to their full potential, have also been discussed.
Figure: Estimating the position of the critical point using the convergence
Another approach is based on the sensitivity of the location of the QCD critical point to the mass of the strange quark ms. By dialing that mass one can bring the critical point to zero or small baryon densities where conventional Monte Carlo methods can be used to track its motion while ms is varied. Surprisingly, the point moves into the wrong direction -- off the QCD phase diagram. This puzzling feature might have several explanations. One possibility discussed recently and at the INT Program, is that the 't Hooft's interaction, responsible for the sensitivity of the location of the critical point to the strange quark mass may not be described correctly by the staggered lattice fermion discretization. The other possibility is that the critical point, if it exists on the QCD phase diagram, is not continuously related to the chiral critical point which can be dialed to zero baryon density by the strange quark mass.
Although the INT Program has focused on theory, the current active interest of experimentalists at RHIC, SPS and future FAIR, has made it a natural venue for a forum for discussions and exchange between the theorists and experimentalists. The week-long INT Workshop embedded into the program has been filled with experimental as well as theoretical talks discussing signatures of the critical point and their theoretical underpinning, as well as experimental strategies and challenges.
Interesting new experimental results have been discussed. For example, several seemingly unrelated quantities (quark-number scaling, multiplicity fluctuations, forward-backward multiplicity correlations, etc.) show a non-trivial centrality dependence. Although it is not yet clear if this is related to the critical point, the workshop provided an opportunity for the discussion and stimulated follow-up measurements that are being carried out now by the RHIC experiments, and will be the focus of future experiments such as NA61/SHINE at CERN SPS.
One of the remarkable examples of synergy between theory and experiment during the workshop has been the emergence of a new set of experimental observables, such as higher order cumulants of fluctuations. Such cumulants have been bread-and-butter quantities for Lattice theorists. Yet, experimentalists studying fluctuations have mostly focused on quadratic, Gaussian, measures of fluctuations. As a result of the discussions at the INT Program the attention of experimentalists at STAR has now turned to observables which measure deviations of fluctuations from Gaussianity.
The question of sensitivity of these higher order cumulants to the proximity of the critical point has also drawn attention of theorists.
The discovery of the critical point would clearly require a concerted effort of theoretical and experimental community. The INT Program and the Workshop served as a fruitful ground for exchange of knowledge and ideas necessary to achieve this goal.
(INT program July 28 - August 22, 2008)
Reported by Volker Koch, Gunther Roland, Misha Stephanov
Date posted November 12, 2008
![]()
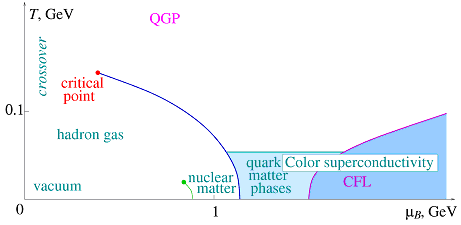
Figure: A sketch of the QCD phase diagram.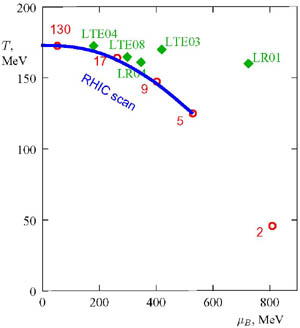
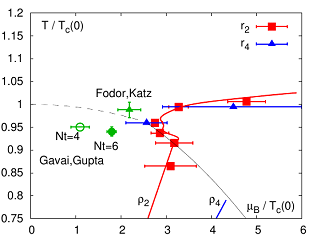
radius of the Taylor expansion in μB. See details in the talk by F. Karsch at the workshop.