Lattice quantum chromodynamics (LQCD), as the only truly ab inito approach for studying hadronic systems, has recently started to address long-standing problems in nuclear physics. Studying nuclear reactions directly from LQCD is the most recent quest in this direction, and amongst the most challenging ones as well. Progress towards this goal will eventually impact our understanding of environments that occurred during big bang nucleosynthesis, within stars and supernovae, and within nuclear reactors and high-energy density facilities. Due to our limited knowledge of some of the most important nuclear reaction cross sections from experiment and their associated large theoretical uncertainties, a direct LQCD calculation of these quantities with well-controlled uncertainties is crucial. Lattice QCD calculations provide a direct input to modern day few-body and many-body nuclear physics calculations, such as no core shell model with continuum (NCSMC) and lattice effective field theory (EFT), and will reduce those systematic uncertainties of these calculations that are due to the poor knowledge of nuclear few-body forces. However, due to limited computational resources for LQCD calculations, as well as the lack of formal development necessary to translate the output of key LQCD calculations to physical quantities, an optimal path toward making the connection between LQCD few-body calculations and nuclear many-body calculations has yet to be identified prior to this workshop. With these challenges and goals in mind, the INT workshop in "Nuclear reactions from Lattice QCD" was held in INT in March 2013 to report on the status of this new field. The progress made by nuclear reaction, EFT, lattice EFT, and LQCD experts regarding this program were discussed extensively, and short-term and long-term goals of the field were established and prioritized at the end of the two-day workshop.

Due to the fact that LQCD calculations are performed in a finite Euclidean spacetime volume, a formalism is needed to connect the lattice QCD outputs to physical quantities of interest. In the two-body sector, the "Lüscher method" presents such a connection [1, 2]. This formalism demonstrates that from the LQCD determination of energy levels of a two-hadron system in a finite volume (FV) one has access to two-body scattering phase shifts at the corresponding energies. Going above the inelastic threshold, where three or more particles are involved in the process, has put major obstacles in interpreting the FV spectrum of multi-hadron systems in recent LQCD calculations. Such formalism is not only crucial in studying nuclear reactions with LQCD, but also in LQCD studies of hadronic resonances. For example, as was discussed in the workshop, the future success of recent JLab LQCD efforts to connect to the upcoming GlueX experiment strongly depends on the development of such formalism. Developments regarding this problem by two independent groups at the University of Washington were presented and discussed in the workshop. One approach, based on non-relativistic EFT, has been used to study a three-body system as an effective 2+1 system and the energy eigenvalues of the system were shown to be related to the physical three-body scattering amplitudes through an integral equation. The implication of this formalism for particle-bound state scattering below inelastic scattering was discussed, and a practical plan for a future LQCD calculation of deuteron-nucleon scattering amplitude below and above breakup threshold was sketched [3]. In another approach, the preliminary result of an investigation of three-body scattering amplitudes below the four-body threshold in connection to the FV spectrum of the system was presented [4]. The workshop presented the opportunity of direct interactions between these two groups as well as other interested participants, and resulted in setting specific plans to be able to compare these seemingly different approaches and reach converging results in this problem.

As an alternative to the well-known Lüscher method, one might aim to access the physical scattering amplitudes by constructing nuclear potentials out of multi-nucleon wavefunctions extracted form LQCD calculations. This potential approach, has been developed and extensively used by the HAL QCD Collaboration [5]. Due to its reference to potentials, which are not physical observables, part of the community questions the validity of such 'scheme'-dependent calculations as compared to the Lüscher method.

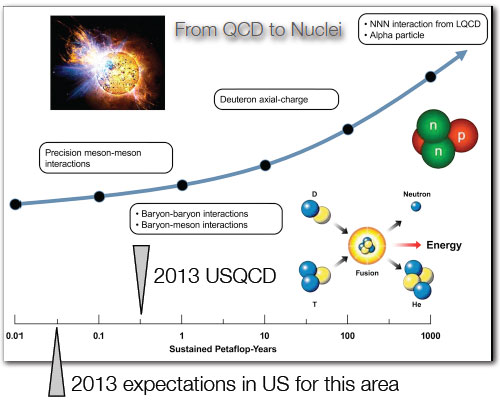
FIG. 1: Anticipated computational resources in terms of sustained Petaflop-years for important calculations needed toward the goal of describing nuclei from the theory of QCD. The current status of USQCD resources, as well as USQCD resources devoted to the nuclear physics program are indicated in the picture. The picture is provided in a report on a "Scientific Grand Challenges" workshop series: Forefront Questions in Nuclear Science and the Role of Computing at the Extreme Scale. The report is available from http://extremecomputing.labworks.org/nuclearphysics/report.stm.

 
Recent formal progress by the HAL QCD in constructing the energy-dependent potentials above inelastic threshold was presented in the workshop [6]. This improvement in the potential method was motivated by discussions in a previous INT program,"Lattice QCD studies of excited resonances and multi-hadron systems " in the summer of 2012. Extensive discussions regarding the pros and cons of such potential approach were continued in this workshop as well.

Given the recent progress in applying EFT chiral forces in nuclear few-body and many body calculations, inputing well-constrained EFT low-energy coefficients (LECs) in these calculations is of great importance. The workshop benefited from a number of experts in EFT many-body calculations who presented a clear path for LQCD practitioners to focus on constraining those LECs that are currently lacking tight constraints in their calculations. The emphasis was put on the need for a precise determination of three and four neutron forces will most likely expedite LQCD determinations of three and four neutron energy levels. These calculations will be still performed at unphysical pion masses (m_π) in the upcoming years, but the recent development in the use of chiral perturbation theory (χPT) to parametrize the nuclear forces in many-body nuclear calculations, such as Quantum Monte Carlo, should facilitate the matching to LQCD results by varying the pion mass in these calculations [7]. Progress in this direction will obviously have great impact in our understanding of neutron rich environments, for example in determining the equation of state of neutron stars which would lead to predictions for gravitational wave experiments. LQCD can also greatly improve the calculations of matrix elements of electroweak interactions. In particular, a novel suggestion was presented and discussed in the workshop to study neutrinoless double-beta decay via LQCD. Another interesting contribution of LQCD in regard to fundamental symmetries would be to determine the WIMP coupling to one and two nucleons for dark matter detection experiments.

The workshop also presented the opportunity of a direct communication between experts in ab initio many-body nuclear reactions and LQCD experts. In particular, the progress in applying the NCSM with resonating group method (NCSM/RGM) as well as NCSMC to nuclear scattering and reactions, [8], was reported and discussed in the workshop. Although NCSMC method has been shown to be successful in describing the ⁷He resonances as well as ⁷Be (p, γ)⁸B radiative capture and ³H (d, n)⁴He fusion using the two-nucleon chiral interactions, a realistic calculation of interesting fusion processes such as n − ⁴He and p − ⁴He requires the inclusion of precise three-nucleon forces [9, 10]. Again LQCD determination of three-nucleon parameters will significantly improve the result of such calculations. It was pointed out in the workshop that quantifying systematic uncertainties due to chiral forces, as opposed to those associated with the many-body techniques used in NCSMC calculations, would give LQCD practitioners a guideline of the level of precision needed for the χPT LECs. Results from Lattice EFT, as another direction of active research in ab initio calculations of nuclear reactions, were presented in this workshop [11, 12]. In addition to constraining the LEC of chiral interactions used in these calculations, LQCD will be able to offer a precise determination of the m_π-dependence of two-nucleon scattering lengths, which have shown to give insight into the long-standing question of how fine-tuned the production of Carbon is with respect to slight variations of the standard model parameters [13, 14].

In summary the workshop presented a great opportunity for an effective communication among approximately 30 participants with expertise in quantum many-body calculations, EFT and LEFT, and LQCD for nuclear and particle physics. As a result of many talks and intensive discussions, the goals were set for the future of the field with
well-defined paths and plans.

[1] M. Lüscher, Commun.Math.Phys. 105, 153 (1986).

[2] M. Lüscher, Nucl.Phys. B354, 531 (1991).

[3] R. A. Briceno and Z. Davoudi (2012), 1212.3398.

[4] M. T. Hansen and S. R. Sharpe (2013), in preparation.

[5] S. Aoki, T. Hatsuda, and N. Ishii, Prog.Theor.Phys. 123, 89 (2010), 0909.5585.

[6] S. Aoki, B. Charron, T. Doi, T. Hatsuda, T. Inoue, et al., Phys.Rev. D87, 034512 (2013), 1212.4896.

[7] A. Gezerlis, I. Tews, E. Epelbaum, S. Gandolfi, K. Hebeler, A. Nogga, and S. Schwenk (2013), 1303.6243.

[8] S. Baroni, P. Navrátil, and S. Quaglioni, Phys. Rev. Lett. 110, 022505 (2013), URL PhysRevLett.110.022505.

[9] P. Navrátil, R. Roth, and S. Quaglioni, Phys.Lett. B704, 379 (2011), 1105.5977.

[10] P. Navrátil and S. Quaglioni, Phys. Rev. Lett. 108, 042503 (2012), URL PhysRevLett.108.042503.

[11] S. Bour, H.-W. Hammer, D. Lee, and U.-G. Meissner, Phys.Rev. C86, 034003 (2012), 1206.1765. [12] G. Rupak and D. Lee (2013), 1302.4158.

[13] E. Epelbaum, H. Krebs, T. A. Lahde, D. Lee, and U.-G. Meissner, Phys. Rev. Lett. 110, 112502 (2013), 1212.4181. [14] E. Epelbaum, H. Krebs, T. A. Lhde, D. Lee, and U.-G. Meiner (2013), 1303.4856.

[15] S. Beane, E. Chang, S. Cohen, W. Detmold, H. Lin, et al., Phys.Rev. D87, 034506 (2013), 1206.5219. [16] T. Yamazaki, K.-i. Ishikawa, Y. Kuramashi, and A. Ukawa, Phys.Rev. D86, 074514 (2012), 1207.4277.