INSTITUTE FOR NUCLEAR THEORY
News
Home | Contact | Search | News archive | Site Map

|
INSTITUTE FOR NUCLEAR THEORY News
Home | Contact | Search | News archive | Site Map |
|||||
Quarks, gluons and nuclear forces
When
students are first
told about the fundamental forces of the Universe, the strong nuclear
force is described as the force binding protons and neutrons together
inside nuclei. Later, they learn about quarks and gluons and the theory
describing them, quantum chromodynamics (QCD). What they do not learn
is how the theory of strong interactions (QCD) actually explains the
strong interactions between nucleons. The reason, of course, is that
nuclear forces are a long distance phenomenon and QCD is notoriously
unyielding to analysis in this regime. In fact, after over 30 years
since its discovery, QCD has provided very few clues regarding nuclear
forces.
Thus we can extract the mass of a particle from the large time behavior
of an euclidean correlator. Similarly, we can extract from the
two-particle correlator the energy levels of a two-particle state. The
bulk of the energy of a two-hadron state is given by the sum of the two
hadron masses. At finite volume though there is a small component
proportional to their interaction: the energy levels go up a bit for
repulsive interactions and down a bit for attractive interactions.
There is in fact a formula (known as Luscher formula) that relates the
shift in the energy level ΔE to the phase shift describing
scattering at energy ΔE. For boxes with size L much larger than
the scattering length a
the Luscher formula reduces to
which is easy to derive
using perturbation theory. The applicability of the method is not
restricted by a/L
<< 1, the only condition is that L be
much larger
than the range of the force between the particles. In particular, the
nuclear s-wave scattering lengths that are fairly large (5-20 fm) can
be studied in boxes just a few fermi wide. In a box of size L ~
2.5 fm the typical values of ΔE is only about 20
MeV and very precise measurements of the energy are then
necessary for a good determination of ΔE and,
consequently, of the phase shifts. Recently a high
statistics calculation reported
an error of only a few percent on the scattering length. Since the
computer power needed for the simulation of light quarks is large,
the values of the quark masses used were larger than the realistic
ones,
corresponding to pion masses in the 300-500 MeV range. An extrapolation
down to realistic quark masses is then necessary for a comparison to
experiments. The use of chiral perturbation
theory is fundamental for that since it provides approximate but model
independent expressions for the dependence of various observables as a
function of the quark mass. In fact, the precision of this calculation
is high enough that special variations of chiral perturbation theory
including discretization effects are used. The result of this
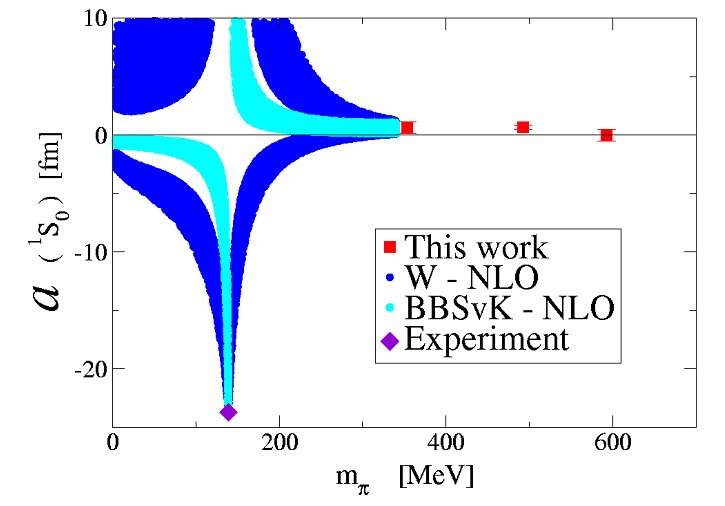
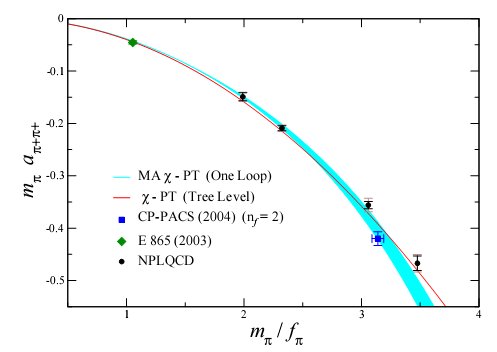
extrapolation is shown in the blue band in the figure together with the
lattice QCD points (in black) and the empirical point in green. Notice that the precision of the
extrapolated point is higher than any individual point at higher quark
masses. This is due to the fact that the scattering length at zero quark
mass, where the pion is a Goldstone bosons, must vanish. Due to this
exact result, the "extrapolation" is actually an interpolation between lattice results and the exact answer at m=0.. Until recently, lattice calculations almost invariably were
done using the quenched calculation. This means that out of the QCD
picture of, for instance, a proton -- three valence quarks surrounded
by many gluons and sea quark-anti-quark pairs -- only the valence
quarks and gluons are kept. Dropping the sea quarks makes it the action
local and reduces the computational cost of the calculation by many
orders of magnitude. For certain observables and for large quark
masses, the quenching approximation is not a huge effect
(~10%). Unfortunately, nucleon-nucleon forces are
strongly changed by quenching and the much more expensive calculations with dynamical sea
quarks are essential.
An effective field theory for nucleon interactions, in the mold
of chiral perturbation theory for pions, exists and has been the
subject of intense scrutiny in the last 10 years. It is, however, much
less effective than it's pionic counterpart. In particular, it suggests
that the s-wave scattering lengths in both spin channels of
nucleon-nucleon scattering will diverge for values of the quark masses
lying between the physical values and the values used up to here.
Clearly, the chiral extrapolation will be a delicate point for this
observable (phase shifts at generic values of momenta do not suffer
from this fine tuning problem and are easier to extrapolate).
Perhaps the most serious problem is that the
signal-to-statistical noise ratio in baryon-baryon correlators decrease
exponentially with (euclidean) time. The rate of decrease
equals 2M-3m
(M is the nucleon mass and m the pion mass) so it is large and it grows
as more realistic pion masses are used. Thus, the statistical noise
renders the information about energy levels residing at large
t useless.
Due mainly to the problem 3) above, the only dynamical calculation
up to now was only able to put bounds on the values of NN scattering
lengths, of the order of 1 fm. This already contains some non trivial
information. It shows, for instance, that the values of the scattering
lengths are indeed fine tuned and modest changes in the quark masses
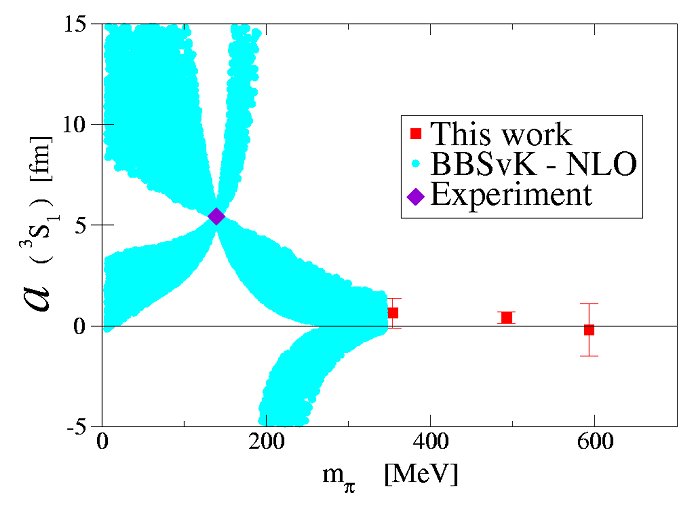
are enough to make them relax to more natural values. Also, using the
knowledge we have about the nuclear effective theories (and the
experimental value of a at realistic quark masses) we can perform the rough extrapolation down in pion masses shown in the figure below.
Further progress of the nuclear physics on the lattice program will require a continued effort along two prongs: Community support: modern lattice calculations resemble large
experiments in some respects. They involve innovations in hardware,
software as well as smoothly running facilities. This can only be
accomplished with the involvement of top experts in different areas of
computer technology.
Innovative ideas: new ideas on how to tackle the problems
unearthed by the pioneering calculations are needed, brute computer
power can't solve everything. These problems are directly linked to the
physics of nuclear interactions and experts in this area -- and not
only lattice experts -- are the most likely to make progress. The use
of chiral perturbation theory/nuclear effective theory in the chiral
extrapolations above is one example of profitable interaction between
lattice and
nuclear community. We can expect that, in the future, experts on
both
nuclear force/few-nucleon physics and lattice practitioners will become
more aware of each others fields and able to contribute to the success
of the program.
Reported by Paulo Bedaque
August 31, 2007
![]()
This is not to say that we don't know much about
nuclear
forces. In fact, we know a lot. If we look at nuclear systems with a
resolution of no more than about 0.5 fermi (corresponding to energies
below the threshold for pion production in nucleon-nucleon scattering),
the complicated dance of quarks and gluons reduces to the much simpler
motion of non-relativistic nucleons interacting through a
(possibly momentum dependent) potential. One can then just take the
attitude that non-relativistic nucleons are the correct degrees of
freedom in nuclei and learn about their interaction in an empirical
way. This point of view was how few-nucleon physics developed even
before the advent of QCD and was more recently systematized by
the effective
field theory approach. In its modern incarnations, the
empirically well known proton-proton and neutron-proton phase shifts are very
well fit by non-relativistic potentials ( AV18, CD-Bonn, Nijmegen
s, ... ) and these potentials are used in the Schroedinger equation for
systems containing several nucleons.
The result is that the empirical potentials provide a good but not
perfect match with what is found in Nature. The discrepancies, found
empirically and predicted/postdicted by the power counting of effective
theory, can be cast as the need for additional terms in the
hamiltonian describing the physics occurring when more than two-nucleons
occupy the same region of space-time. Examples include a three-nucleon
force and two-nucleon-photon or two-nucleon-neutrino contact terms.
These terms are, obviously, not constrained by nucleon-nucleon data.
The problem is that the data that can constrain them are,
frequently, very hard to measure. This problem is particularly acute in
the extension of this approach to the study of hyperon interactions
where the available data is nowhere as complete as
in the nucleon-nucleon case (and will never be).
We see then that, besides the conceptually important goal of a "first
principles" understanding of nuclear binding,
there are other, more "practical" reasons to hope for a QCD-based
approach to nuclear interactions. Currently, the only general purpose
approach to QCD in the non-perturbative regime is lattice QCD. QCD,
like any field theory, can be defined by a functional integral over the
fields describing gluons and quarks. This is nothing but the field
theoretical version of Feynman's "sum over histories" in quantum
mechanics. The basic idea
of lattice QCD is to discretize space-time - so the functional integral
becomes an integral in a space with a large but finite number of dimensions - and calculate the integral
by Monte Carlo methods. Although straightforward in principle, this
program is enormously difficult in practice, even when considering much
simpler observables like stable hadron masses. In the last few
years a number of advancements came together to make the promise of
phenomenologically useful lattice calculations a reality. To the
outsider, the most obvious progress is the increase of the sheer power
of computers, be them special purpose machines (like QCDOC)
or clusters
of commodity pc's dedicated to lattice calculations. But equally
important
is the development in algorithms and innovative approaches to lattice
calculations like the use of quark discretizations with exact chiral
symmetry and, specially, the use of improved action
discretizations. What is "improved" in these discretizations is the
approach to the continuum limit: if the space-time lattice
spacing is a,
naive discretizations will have an error of order a Λ
, where Λ is a typical QCD scale (say ~300 MeV). Improved
actions will have smaller errors, scaling like (a Λ)2.
This improvement allows for precise results to come out even of rather
coarse lattices (typical lattices nowadays have a of order of 0.1
fm). The saving in computer requirements is huge.
All these improvements help the lattice calculation of any observable. But the study of
nuclear forces -- or hadron interactions in general -- has its own
specific difficulties requiring specific solutions. Until about a
year ago, the only study of nuclear forces in lattice QCD was a 1995
pioneer quenched calculation,
and only recently dynamical, exploratory calculations started telling us what the
real issues are. Let us consider two recent results of the NPLQCD
(Nuclear
Physics with Lattice QCD) collaboration.
The first, a warm up
problem with its own physics interest, is the ππ
scattering in
the I=2 channel. Since lattice QCD calculations are done in imaginary
time, scattering amplitudes are not directly accessible. The way NPLQCD
chose to extract scattering information was the use of the "Luscher method".
It is based on the observation that while scattering amplitudes cannot
be extracted from euclidean correlators, energy levels can.
For instance, if π(t,r) is some operator with pion quantum
numbers, |n> are energy eigenstates and |π> a one
pion at rest state we have
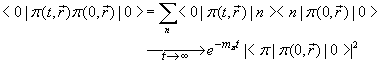
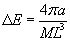
Several factors make the lattice study of nucleon-nucleon interactions
more difficult than the ππ case: