INSTITUTE FOR NUCLEAR THEORY
News
Home | Contact | Search | News archive | Site Map

|
INSTITUTE FOR NUCLEAR THEORY News
Home | Contact | Search | News archive | Site Map |
|||||
From Femtoscience to Nanoscience: Nuclei, Quantum Dots and Nanostructures
The program was interdisciplinary in nature and brought together the nuclear and condensed matter communities. Its main focus was on correlation effects in finite-size quantum systems such as nuclei, quantum dots and nanostructures.
We had a total of 48 talks. A few of these talks were by leading experimentalists who presented state-of-the-art overviews of their fields. There were numerous fruitful interactions between participants within and across different disciplines. The program benefited from active participation by both nuclear and condensed matter theorists from the INT and the Physics Department at the University of Washington.
The program demonstrated that the nuclear physics and mesoscopic physics communities share similar interests and methods. One of the challenges in nuclear theory is the inclusion of many-body correlations beyond the mean-field approximation. Similarly, the single-particle degrees of freedom of mesoscopic electronic structures are well understood now, and interest has shifted towards understanding the role of many-body correlations.
The program led to significant progress on a broad range of topics. We summarize below some of these advances.
Pairing correlations in finite-size systems. The Bardeen-Cooper-Schrieffer (BCS) theory is the standard mean-field approximation to describe the effects of pairing correlations but it breaks down when the bulk gap Δ becomes comparable to the single-particle mean-level spacing δ. This is the case in both nano-scale metallic grains and in nuclei. Various strategies to account for pairing correlations beyond BCS theory were discussed.
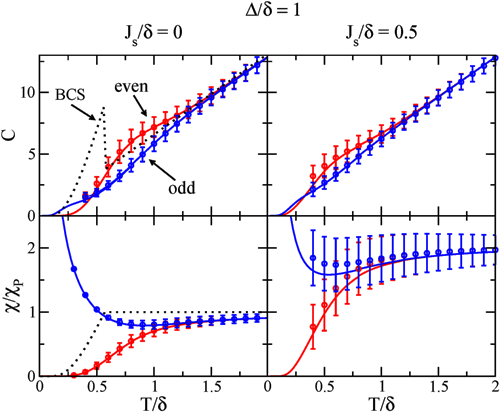
The low-energy physics of a mesoscopic chaotic structure is characterized by an effective Hamiltonian known as the universal Hamiltonian. The universal Hamiltonian describes the competition between pairing correlations, which favor a superconducting minimal spin ground-state, and the exchange interaction, which favors a ferromagnetic spin-polarized state. Signatures of this competition can also be identified in the thermodynamic properties of the grain. We discussed methods to calculate such properties in which pairing correlations are accounted for by large-amplitude static fluctuations plus small-amplitude time-dependent fluctuations of the pairing field, while the exchange interaction is treated exactly. Figure 1 shows the heat capacity and spin susceptibility of an ultra-small grain with a gap of Δ/δ=1. Similar number-parity effects induced by pairing correlations were found in the heat capacity and moment of inertia of nuclei.
Fig. 1: Thermodynamic observables (versus temperature T) of a nano-scale metallic grain with a pairing gap of Δ/δ=1: heat capacity C (top panels) and spin susceptibility χ in units of the Pauli susceptibility χp (bottom panels). Results are shown in red (blue) for a grain with even (odd) number of electrons. Circles and vertical bars describe, respectively, the average and standard deviation of the mesoscopic fluctuations, while solid lines correspond to an equally-spaced single-particle spectrum. Left panels: no exchange correlations (the dotted lines describe the BCS approximation). Right panels: exchange correlations are included with a coupling strength of Js/ δ=0.5. Exchange correlations suppress number-parity effects in the heat capacity, which are induced by pairing correlations, but enhance the mesoscopic fluctuations of the spin susceptibility (K. Nesterov and Y. Alhassid).
In nuclei, pairing correlations lead to odd-even staggering of nuclear masses. There have been recent microscopic studies of the global systematics of this effect that are based on density functional theory with a BCS treatment of the pairing interaction. We discussed methods to improve the agreement with experimental results by replacing the BCS pairing correlation energy with the exact pairing correlation energy.
Integrable models that generalize the Richardson solution of a BCS-like Hamiltonian were discussed in the context of nuclear models that conserve isospin and of models of metallic grains that describe the competition between superconductivity and ferromagnetism.
Quantum chaos in many-particle systems. Finite interacting fermionic systems such as nuclei display statistical fluctuation properties that can often be characterized by random matrix theory, and are commonly referred to as many-body quantum chaos. Weakly interacting electrons in disordered conductors undergo a metal-to-insulator transition that can be understood as an Anderson-like localization of the many-particle wave function in Fock space. An analogy to this metal-insulator transition was discussed in the study of delocalization and chaos in random-matrix models of interacting fermions. The random matrices describing such models are sparse. The question of whether their eigenvalues follow Wigner-Dyson statistics was answered affirmatively by constructing random-matrix ensembles that are even more sparse and have similar statistical properties.
Interacting many-body systems in one dimension. Interaction between particles confined to one dimension (1D) changes their behavior qualitatively: low-energy excitations of a fermionic 1D system cannot be described by almost-free fermionic quasi-particles, and even weak repulsion destroys the 1D Bose condensate. At the lowest energy scales, the conventional Tomonaga-Luttinger model provides a satisfactory description of such 1D systems. However, recent experiments with quantum wires and trapped cold atoms demonstrate the need for a theory that is applicable at higher energies. The developments discussed during the program include the use of integrable models, exploiting conservation laws and kinetic theory ideas, and using analogies with the Fermi edge singularity problem.
It is commonly accepted that there are no phase transitions in 1D systems at a finite temperature because long-range correlations are destroyed by thermal fluctuations. An interesting development in the program was the demonstration that a 1D gas of short-range interacting bosons in the presence of disorder can undergo a finite-temperature phase transition between a fluid state and an insulator state. This phase transition is non-conventional in the sense that there are no long-range spatial correlations but the transport properties are singular at the transition point. Mass transport is possible in the fluid phase but is completely blocked in the insulator phase. We therefore revealed how the interaction between disordered bosons affects their Anderson localization. This central issue, first raised for electrons in solids, is now crucial for studies of atomic bosons, where recent experiments have demonstrated Anderson localization in expanding dilute quasi-1D clouds.
Graphene. Graphene, a single atomic layer of graphite, is a gapless semiconductor whose charge carriers mimic relativistic massless Dirac fermions as dictated by the highly symmetric honeycomb lattice of the crystal. Recently, it has become possible to measure the transport properties of graphene quantum dots. This experimental progress was discussed in the program and opens the possibility of studying the mesoscopic properties of such structures.
The electronic transport properties of graphene can be strongly affected by atoms adsorbed on its surface (adatoms). Often such adatoms assume random positions in the graphene lattice and we discussed the possibility of formation of a correlated state. Partial ordering of adatoms that leads to a gapped spectrum was identified. The character of the partial ordering transition depends on the position of the adatoms. When the adatoms are located in the middle of the hexagon of the honeycomb lattice or on the bond between two closest carbon atoms, there is a tendency to form a spatially correlated state with a hidden order. However, when the adsorbents are attached to the lattice sites, the ordering transition consists of the preferential occupancy of one sublattice by all adatoms in a dilute ensemble.
"Open" interacting Fermi systems. There are analogies between nuclear reaction processes and transport through a quantum dot. In both cases an interacting Fermi system is coupled to the continuum. Several methods exist to calculate the transmission through an open or an almost-closed dot, but the case of a semi-open dot remains an outstanding issue. We discussed a method in which the degrees of freedom of the leads are integrated out exactly. The difficulty is in treating the resulting effective action of the dot, which becomes non-local in time.
In conclusion, our interdisciplinary program provided a unique opportunity for nuclear physicists to interact with condensed matter physicists on topics of joint interest. The exchange of ideas stimulated numerous collaborations and led to substantial progress in both fields.
(INT program July 20 - August 28, 2009)
Reported by Y. Alhassid (Yale University), B.L. Altshuler (Columbia University), and V.I. Fal'ko (Lancaster University, UK)
Date posted March 11, 2010
![]()